|
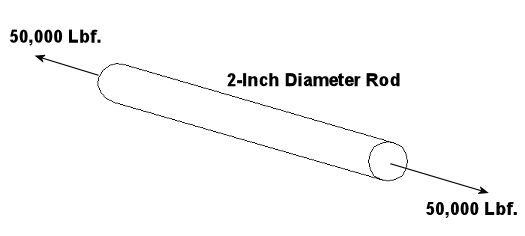
When we looked at our statics example a few posts ago, it was convenient to consider the bridge beam as being perfectly rigid. In the real world, a bridge beam is not perfectly rigid. When it’s subjected to external forces, like the weight of a truck sitting in the middle of it, there is a tendency for it to bend, deform, change its shape. If the external forces create bending and deformations that are too much for the bridge beam material to handle, it will collapse. In strengths of materials analysis, engineers must consider typical stresses that will occur within objects like bridge beams, airplane wings, bolts, and machine parts during use. Stress is a measure of force exerted per unit area of a surface. Once the stresses are known, a material is selected that is strong enough to keep the object from being bent, mashed, stretched, and/or broken. To illustrate this, let’s consider an example of a simple stress. Suppose you want to design a truss rod for a highway bridge like the one shown in Figure 1. Specifications require that the rod be two inches in diameter. Its purpose is to hold the sides of the bridge together when vehicles travel across it. Figure 1 Now suppose that the bridge is to handle traffic to and from and iron mine. Let’s consider the scenario where a truck laden with iron ore runs out of gas and comes to a stop in the middle of our bridge. Under this load, static analysis reveals that the sides of the bridge want to spread apart, putting a tension of 50,000 pounds force (Lbf.) on the truss rod as shown in Figure 2. Figure 2 Since the external force acting upon the truss rod and the diameter of the rod itself are both given, the engineer would merely have to select the appropriate material for the rod that would fall within the desired parameters, that is, so as to keep our rod from pulling apart when anticipated stressors such as a heavy truck is on the bridge. The first step would be to calculate the tensile stress within the rod itself. By tensile stress, I mean the stress in the rod due to the forces that are trying to pull it apart. In our example, this is relatively easy to assess: Stress = [Tensile Force] ÷ [Lateral Cross Sectional Area of the Rod] The lateral cross section of the rod is round, so its area would be πd2 divided by 4, where the Greek letter π (Pi) has a value of 3.1416, and d is the diameter of the rod. Therefore, the tensile stress would be: Stress = [50,000 Lbf.] ÷ [π × (2 in.)2 ÷ 4] = 15,915.49 Lbf./in.2 Knowing the stress within the rod, the engineer would next have to select an appropriate material for the rod that’s strong enough to do the job. In our case, let’s say we determine that steel is the best material to use. But what type of steel? Well, there are engineering handbooks with tables that list the mechanical properties of all sorts of materials, including metals and plastics, and along with those properties there is listed their ability to handle stress. These mechanical properties were determined in laboratory tests where carefully machined specimens of the materials were subjected to measured stresses until they deformed and broke apart. They include yield strength and ultimate strength, and these findings have been duly recorded so that future engineers need not go through independent testing of common materials themselves. Yield strength is the stress that is measured when the test specimen begins to stretch without any significant increase in force being applied to it. Ultimate strength is the maximum stress that the material in the test specimen can withstand before it starts to fail, that is tear apart, break, collapse. Our engineer would use the mechanical properties tables that are readily available to him to select the appropriate steel alloy that would meet our criteria, that is, has a listed strength high enough above the calculated 15,915.49 Lbf./in.2 tensile stress to provide a sufficient factor of safety. Factors of safety are listed in engineering books for yield strength and ultimate strength and they are selected depending on how the forces are applied to a structural component or machine part (e.g. steady, varying, or shock). When strengths of materials analysis involves the solution of complex statics and dynamics problems, things become a little less straightforward. And when you are dealing with components having odd shapes and a combination of stresses, say due to compression and torsion, things become even more complex. In any case, engineers must have extensive knowledge of the properties of materials in order to anticipate factors at play in real world scenarios such as the one we’ve been discussing. Ever wonder why some steel is hard and brittle, and some is soft and bendable? Well, our next topic of discussion will be about materials science, the study of how metal alloys and polymers are formed to have specific properties. _________________________________________________________________ |
Posts Tagged ‘dynamics’
Strengths of Materials in Mechanical Engineering
Sunday, November 15th, 2009Mechanical Engineering, Focus on Dynamics, Part II, Kinetics
Monday, November 2nd, 2009|
In Part I of Mechanical Engineering, Focus On Dynamics, we talked about kinematics. This week in Part II, we’ll talk about kinetics. Kinetics is the study of the relationship that exists between forces acting on a body, the mass of the body itself, and its motion. It’s used to predict the motion caused by given forces acting on a body or to determine the forces required to produce a motion of the body. In kinetics forces can be attributed to the motion of an object by way of Sir Isaac Newton’s Second Law of Motion. In other words, this law relates the kinematics of an object to the forces applied to it, and in equation form that relationship looks like this: Force = [Mass] x [Acceleration] “Mass” is the weight of the object, in pounds, divided by the acceleration of the Earth’s gravity. On Earth, the acceleration of gravity is considered to be a constant and is taken to be 32.2 feet per second, per second. (No, that’s not a typo.) Legend has it that Newton discovered the earth’s acceleration when gravity caused an apple to fall from a tree and hit him on the head. That may or may not be true, but Newton’s Second Law of Motion forms the foundation for the study of kinetics. To illustrate how kinetics works, we can look at another very simple example. Suppose you have a block of steel that weighs 50 pounds, and it is sitting still on a nice smooth, frictionless sheet of ice (see Figure 1). Figure 1
How hard would you have to push on the side of this block to make it accelerate across the ice at a rate of 10 feet per second, per second? Use Newton’s second law to find out: Force = [(50 Lb.) ÷ (32.2 ft./second2)] x [10 ft./second2] Force = 15.5 Lb. Force and energy calculations in kinetics can get really complicated, especially when you have to consider the effects of friction, forces acting at angles, velocity, rotation, motion constraints, collisions, etc. Our next mechanical engineering topic will be: Kinematics of Machines, the study of how parts of machines behave as they move through their ranges of motion. _________________________________________________________________ |
Mechanical Engineering, Focus on Dynamics – Part I, Kinematics
Sunday, October 25th, 2009|
Last week we talked about one of the ten core disciplines of mechanical engineering, statics. Today our focus will shift to a discussion of how mechanical engineers use the study of Dynamics to solve problems. As its name implies, Dynamics involves the study of how things change, or more specifically, how they move. Dynamics can be broken down into two basic parts: kinematics and kinetics. We’ll focus on kinematics this week. Kinematics is the study of the geometry of motion. Kinematics is used to develop a relationship between how far an object travels in a given time, its velocity, and its acceleration. The precipitating cause of the motion is not considered in kinematics. Before beginning our discussion of kinematics, let’s go back to the good ol’ days and revisit what should be some very familiar ground to anyone who’s taken algebra. Does this word problem sound familiar to you: If you start at point X and you’re going at Y speed for a given length of time, T, what distance will you have traveled? Here’s the same problem presented in another way. Suppose you’re driving and you set your cruise control at 70 miles per hour. Out of curiosity you want to know how far your automobile will travel in 15 minutes (0.25 hours). Kinematics teaches that the relationship between velocity, time, and distance can be demonstrated by this equation: Distance = (Velocity) x (Travel Time) Distance = (70 miles/hour) x (0.25 hour) = 17.5 miles Of course kinematics can get far more complicated than this simple example, especially when objects accelerate, decelerate, change direction, and move in paths that are nonlinear, that is, not in a straight line. Next week we’ll talk about the study of kinetics, particularly Sir Isaac Newton’s Second Law of Motion. _________________________________________________________________ |