|
Last time we learned how Henry Cavendish used Christiaan Huygens’ work with pendulums to determine the value of g, the acceleration of gravity factor for Earth, to be 32.3 ft/sec2, or 9.8 m/sec2. From there Cavendish was able to go on and arrive at values for other factors in Isaac Newton’s gravity formula, namely G, the universal gravitational constant, and M, Earth’s mass. Today, we’ll discuss how Cavendish was able to calculate the Earth’s mass. Newton’s formula for gravity, once again, is: M = (g × R2) ÷ G where M stands for the mass of the heavenly body being quantified. For our case today M will represent the mass of Earth, which was originally quantified in slugs, a British unit of measurement. Today the measurement unit of choice in most parts of the world is the kilogram, which is the metric equivalent of a slug. With regard to the other variables in Newton’s gravity formula, namely, R and G, their values had previously been determined. Eratosthenes’ measurement of shadows cast by the sun on Earth’s surface had revealed Earth’s radius, R, to be 6,371 kilometers, or 6,371,000 meters. And Cavendish’s experiments led him to conclude that the universal gravitational constant, G, was 6.67 × 10-11 cubic meters per kilogram-second squared. Plugging these values into Newton’s equation, we calculate Earth’s mass to be: M = ((9.8 m/sec2) × (6,371,000 m)2) ÷ (6.67 × 10-11 m3/kg-sec2) M = 5.96 × 1024 kilograms Incidentally, 5.96 × 1024 is scientific notation, or mathematical shorthand, for the number 5,960,000,000,000,000,000,000,000. That’s a whole lot of zeros! Calculating the mass of Earth was an impressive accomplishment. Now that its value was known, scientists would be able to calculate the mass and acceleration of gravity for any heavenly body in the universe. We’ll see how that’s done next time.
_______________________________________
|
Posts Tagged ‘Christiaan Huygens’
What is Earth’s Mass?
Friday, November 7th, 2014
How Long is a Pendulum’s Swing?
Wednesday, October 29th, 2014|
What would you do to pass the time if you were stuck on a ship of the middle ages for weeks at a time? Dutch mathematician Christiaan Huygens used the time to study the movement of clock pendulums. He watched them for endless hours, and he eventually came to realize that the pendulums’ swing was uneven due to the ship’s listing on the waves, a phenomenon which also affected the ship’s clocks’ accuracy. Eager to devise a solution to the problem of inaccurate time keeping, Huygens dedicated himself to finding a solution to the problem, and in so doing increase the navigational accuracy of ships as well. His efforts eventually resulted in a formula that shared a common variable with Isaac Newton’s gravitational formula, namely, g, Earth’s acceleration of gravity factor, a value which Huygens posited was indeed a non varying constant.
Building upon Newton’s work, Huygens devised a formula which demonstrated the mathematical relationship between the motion of a clock’s pendulum and g. That formula is, T = 2 × ∏ × (L ÷ g)1/2 where, T is the period of time it takes a pendulum to make one complete swing, ∏ the Greek symbol pi, valued at 3.14, and L the length of the pendulum. Since devices capable of directly measuring the Earth’s gravity did not exist then, as they still don’t exist today, how in the world (pardon the pun) was Huygens able to arrive at this formula? Thinking outside the box, he posited that if one knows the length of the pendulum L, and then accurately measures the time it takes for the pendulum to complete its swings, taking into account the varied times that resulted due to the ship’s listing, one can calculate g using his equation. He eventually determined g‘s value to be equal to 32.2 feet per second per second, or 32.2 ft/sec2. Fast forwarding to Henry Cavendish’s time, Huygens’ work with pendulums and his determination of g was well known. We’ll see what Cavendish did with this knowledge next time.
_______________________________________
|
Huygens’ Use of Pendulums
Tuesday, October 21st, 2014|
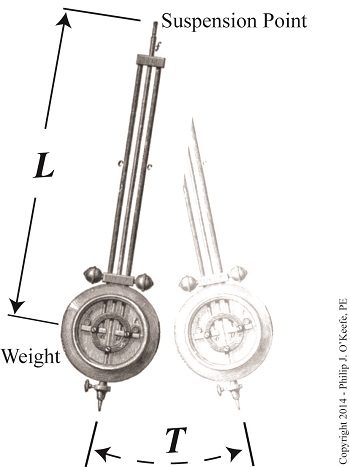
Last time we learned that Henry Cavendish determined a value for G, the universal gravitational constant, fast on his way to determining a quantity he was determined to find, the Earth’s mass. Today we’ll see how the previous work of Christiaan Huygens, a contemporary of Isaac Newton’s, helped him get there. First Cavendish used algebra to rearrange terms in Newton’s gravitational formula so as to solve for M, Earth’s mass. Rearranged, Newton’s formula becomes, M = (g × R2) ÷ G But in order to solve for M, Cavendish first needed to know Earth’s acceleration of gravity, g. To aid him in this calculation he referred back to the work of Christiaan Huygens, a Dutch mathematician from Newton’s time.
Huygens was eager to devise a formula capable of predicting clock pendulums’ motions on ships, his goal being to invent a timepiece accurate enough to make navigating ships easier. He hypothesized that a key factor in predicting a pendulum’s movement was an unknown constant, the acceleration of gravity factor, g, which Newton had previously posited existed. Through meticulous observation, Huygens came to realize that the time it took for pendulums to complete one swing back and forth was dependent not only on the length of the pendulum, but also this unknown quantity. In order for Huygens’ computations to work, the value of g had to be a constant, meaning, its value could not vary between computations; g‘s value was in fact a fudge factor, a phantom he would assign a specific numerical value. Huygens’ needed it in order to make his hypothesis work, a practice commonly use by scientists, even today. Determining a value for g would allow Huygens to successfully relate the length of the pendulum to the timing of its swing and to create a mathematical relationship between them. Huygens ultimately determined g’s value to be a whopping 32.2 feet per second per second, or 32.2 ft/sec2. We’ll see how he did it next time.
_______________________________________
|
